|
Photographs:
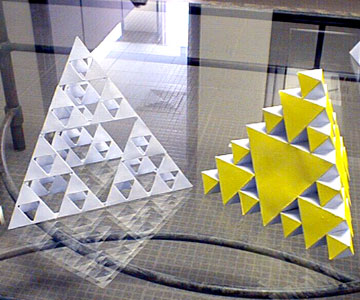
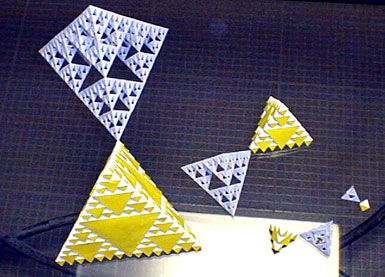
Sierpinski Tetrahedron and its Complement
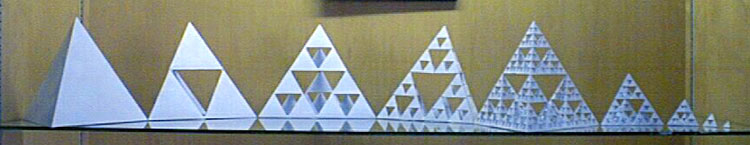
Two Sierpinski tetrahedron families sitting side-by-side with a shared stage-4 structure.
|
|
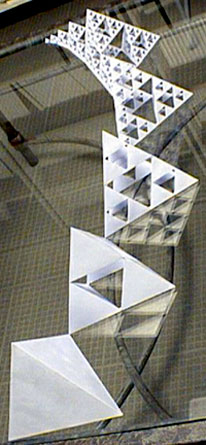
The above photograph shows two Sierpinski tetrahedron
families from dual growth perspectives.
The left arc shows
3R: Reduce-Replicate-Rebuild
growth. The right arc shows 2R: Replicate-Rebuild
growth. The most intricate structure is
shared--it belongs to both families and is at the
cusp where the arcs meet. The starting shape (initiator)
for the Sierpinski tetrahedron is a tetrahedron,
and it grows according to a rule
(generator) whereby each tetrahedron is replaced in the next stage by four tetrahedra
set tip-to-tip. The Sierpinski tetrahedron,
like other geometric fractals,
grows either by using a diminishing initiator that
shrinks by a scaling factor at each stage, or a constant initiator
that stays the same size. |
|
|
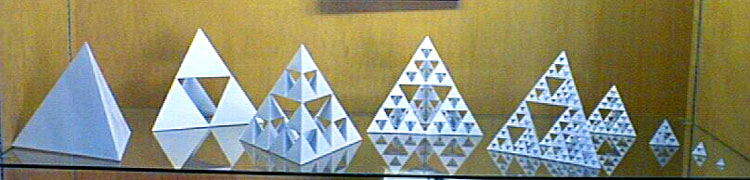
Each family begins with a single tetrahedron, the stage-0. Far-left and moving right with the
3R family, the stage-0 was
Reduced in edge length by one half,
Replicated four times and Rebuilt to form the stage-1, the stage-1 was Reduced in edge-length by one half, Replicated four
times and Rebuilt to form the stage-2, etc. However,
starting far right and moving left with the 2R
family, the stage-0 was Replicated as-is four times and Rebuilt to form a stage-1
with double the edge length of the stage-0, then the stage-1 was Replicated as-is four times and Rebuilt
to form a stage-2 with double the edge-length of the stage-1, etc. |
|
When looking at the structures,
notice how the parts are similar to the whole, called
similarity, a mathematical property
fundamental to fractals. Self-similarity, possible
only for geometric fractals, is achieved only
at infinity when every tiny part is identical to the whole, something not shown or
otherwise approximated here. Approximations of self-similarity are made using
computer programs and are defined by an inability to distinguish
between iterations on the computer screen.
|

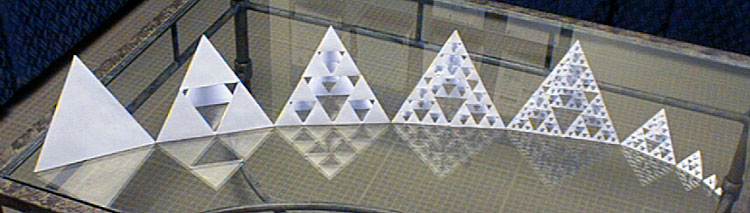
stage-0's, no openings
all volume intact
|

stage-1's, 1 opening
1/2 volume left
|

stage-2's, 2 sizes of openings
1/4 volume left
|
|
The rows of images directly above and below show the structures separated
by same-stages. There is a huge disparity in relative size between the stage-0's.
This disparity decreases until they come together (in size) exactly and only in the stage-4 structure. The choice of where
the structures would intersect was arbitrary; I chose the stage-4 to be
the shared structure. |
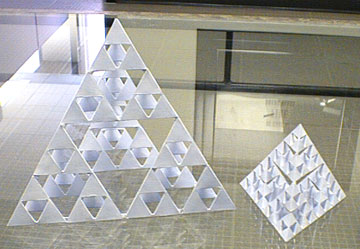
stage-3's, 3 sizes of openings
1/8 volume remains
|
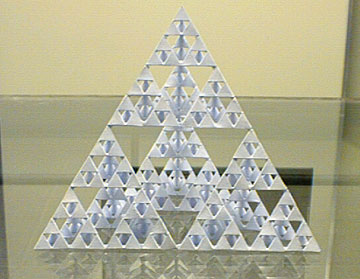
stage-4, count the sizes of openings
1/16 volume remains
|
|
One way to recognize what stage (or level) a structure is, is to
start at the top-oriented vertice and draw a line straight down the center
of a front-oriented face ending at the bottom-center of that same face, and count the number
of openings passed across. It will be one for the stage-1, two for the
stage-2, etc. Another way to determine the stage is to count the number of different sizes of
openings.
|

|
|
|
The number of tetrahedra in any given stage are
increasing by multiples of four. The stage-0 is one (1) tetrahedron, the stage-1
has 1x4=4 tetrahedra. The stage-2 has 4x4=16 tetrahedra. The
number of tetrahedra at any given stage, or iteration, is always the
number of tetrahedra in the previous iteration, multiplied by four. This is exponential growth in powers of 4.
At any given stage of growth,
the Sierpinski tetrahedron is made up of
4N tetrahedra, where N is number of the stage. The stage-5, for instance,
has 45=1,024 tetrahedra. The stage-14 has 414=268,435,456 tetrahedra set
tip-to-tip!
|
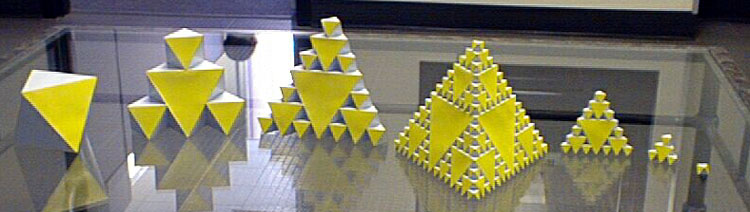
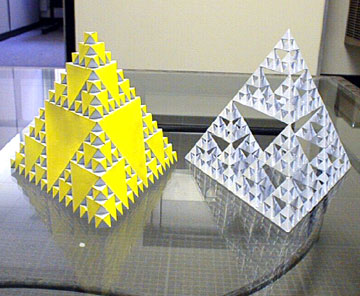
The Complements are a representation of the volume
being removed from Sierpinski's tetrahedron in iterative stages.
|
|
The image of yellow structures directly above are
Complements (aka "negative" aka "inverse" aka "subtraction structures") of Sierpinski's tetrahedron, meaning what is no longer
a part of it at a given stage of growth. The Complement plays a role
similar to that of a negative to its corresponding photograph.
Each stage of the Sierpinski tetrahedron,
when combined with its Complement, comprises a solid tetrahedron. The parts
of the Complement that would be showing through the spaces in the
tetrahedrons if it were sitting inside have been painted in yellow.
|
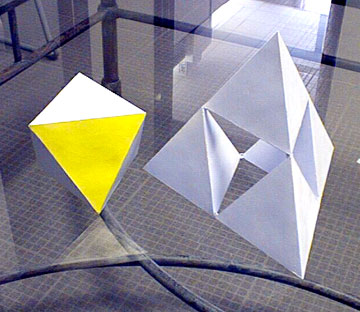
stage-1 complement:
an octahedron
|
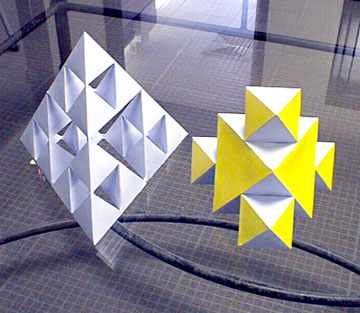
stage-2 complement: made of octahedra
but outer shape is no longer an octahedron
|
|
The Complement starts out as a single octahedron and
is made up of increasing numbers of octahedra.
When the initial octahedron is removed from the stage-1,
it takes half the volume. The next
iteration takes another half, leaving only 1/4 of the original
volume, and the next step (see below left) takes another 1/2 of the volume,
leaving only 1/8 of the original volume! Each stage takes half again of
what was left at the previous stage, and all of that shows up in the
Complement. The stage-4 Sierpinski
tetrahedron (below-right) has
only 1/16 remaining of the original volume
of the solid stage-0.
Collectively, the growing cluster of octahedra that comprises
the Complement is moving toward the shape of a
tetrahedron, not a
surprize, since it is a tetrahedron that is being emptied!
|
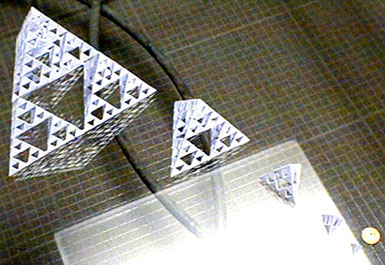
stage-3 Sierpinski tetrahedron and complement,
what shape is evolving in the complement?
|
as we empty a tetrahedron,
the complement is becoming one!
|
|
Even though the Complement represents the emptied volume of an evolving fractal, since it starts
out in the shape of an octahedron and becomes an entirely different shape--a tetrahedron--it is
automatically precluded from being a fractal. Question: Why wasn't
a picture of the stage-0 Sierpinski Tetrahedron and its
Complement included before the stage-1 two rows up? (Answer provided at bottom of page.)
|

|
|
Beginning with the stage-4 Sierpinski tetrahedron,
there is hidden volume in the shape of tetrahedra contained in the interior of the Complement,
exactly 24 for the stage-4. I believe there are 240 interior tetrahedra in the stage-5
Complement, and 1,560 interior tetrahedra in the stage-6 Complement, but I'm not sure about these numbers.
While they are definitely there and increasing in number
rapidly, the interior tetrahedra contained inside the Complement are becoming relative specks, in effect
contributing to a ribbon of volume that will define the Sierpinski Tetrahedron as it approaches
infinity in what is mathematically referred to as "the Limit." I built the Complements from scratch in order to see what they looked like, unable to form an accurate mental picture without something tangible to look at.
I've put up these images
for observation and healthy speculation, also to draw attention to them, and finally because looking at them
and thinking about their implications lends perspective to the process that is taking place
in the Sierpinski tetrahedron as it evolves.
|

|
|
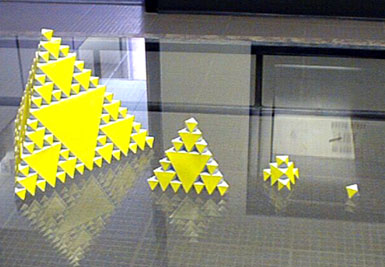
What would the stage-5 structures look like
that would accompany the two families
shown on this page?
There would have
to be two stage-5's.
Consider first the Reduce-Replicate-Rebuild (3R) same-size family,
and let that arc continue along its path instead of ending at the stage-4.
The stage-4 structure at the far right in the above picture would have to
be Reduced, Replicated, and Rebuilt
tip-to-tip to make a stage-5 structure
with four times the number of tetrahedra of the stage-4: 45=1,024 tetrahedra,
each with 1/4-inch edge-length, maintaining the overall edge-length of the structure at 8-inches,
far too tiny and intricate for me to build accurately by hand. [As an aside, a rapid prototyping machine could
produce a beautiful 8-inch stage-5 model, it could likely produce a beautiful 8-inch stage-10 model,
but it would cost a fortune to do it. A friend of mine has even talked of the
possibility of making microscopic!!! Platonic solids using this technology.]
|
|
|
|
The Replicate-Rebuild (2R)
stage-5 structure
would have double the edge-length of the stage-4, built from four full-size
copies of the stage-4 structure set tip-to-tip. Maintaining
a scale of 1/2-inch edge-length for the tetrahedra, it would have an overall edge-length of 16
inches, still a reasonable
size to carry around.
This stage-5 could be accurately built by hand and be a useful structure.
The stage-6, however, would be size-prohibitive. With 46=4,096 tetrahedra and an
overall edge-length of 32 inches, it would be too big and delicate
to even carry in a car! Even though the size of the individual tetrahedra in this model of
growth is held constant, it is the relationship
of volume to removed volume that matters here, and that important relationship is
identiallly preserved in both families.
|
|

|
|
If you are interested in learning more about fractals,
you should consider visiting the Frame-Mandelbrot-Neger website at
http://classes.yale.edu/Fractals.
They also have a lengthy selection of activity labs with visual and written instructions
(including a lab on the Sierpinski tetrahedron) at http://classes.yale.edu/Fractals/Labs/.
Some of the labs, such as Fingerpaint Fractals, use only paper and fingerpaints,
and are appropriate for younger grades. I have referenced two growth explanations here:
1) the 3R/2R growth explanation that runs throughout the page comes from an NSF Pattern
Exploration Institute run by Heinz-Otto Peitgen and Richard F. Voss at Florida Atlantic
University, Boca Raton campus, and 2) the initiator/generator
explanation of fractal growth addressed in the first paragraph comes from Mandelbrot's
Fractal Geometry of Nature. |
|
|
Answer to question above:
There is no image shown for
the stage-0 Sierpinski Tetrahedron with its Complement
because there is no Complement for the stage-0. It is a solid
tetrahedron. Notice both the stage-0's sitting alone in the top-left corner of the
picture directly below. They are there because I didn't want to leave
them out of the picture, but they are without Complement structures
because no volume has been removed from them.
|
|
Okay, and here may be the most beautiful work with the Sierpinski Tetrahedron that
I have ever seen, out of Japan--unfortunately, there are only a few words to read but the pictures are instructive--the work of
Hideki Tsuiki, and here is his
homepage.
|
|